Area = ½ × (c) × (b × sin a) which can be simplified to: The triangle area formula is:
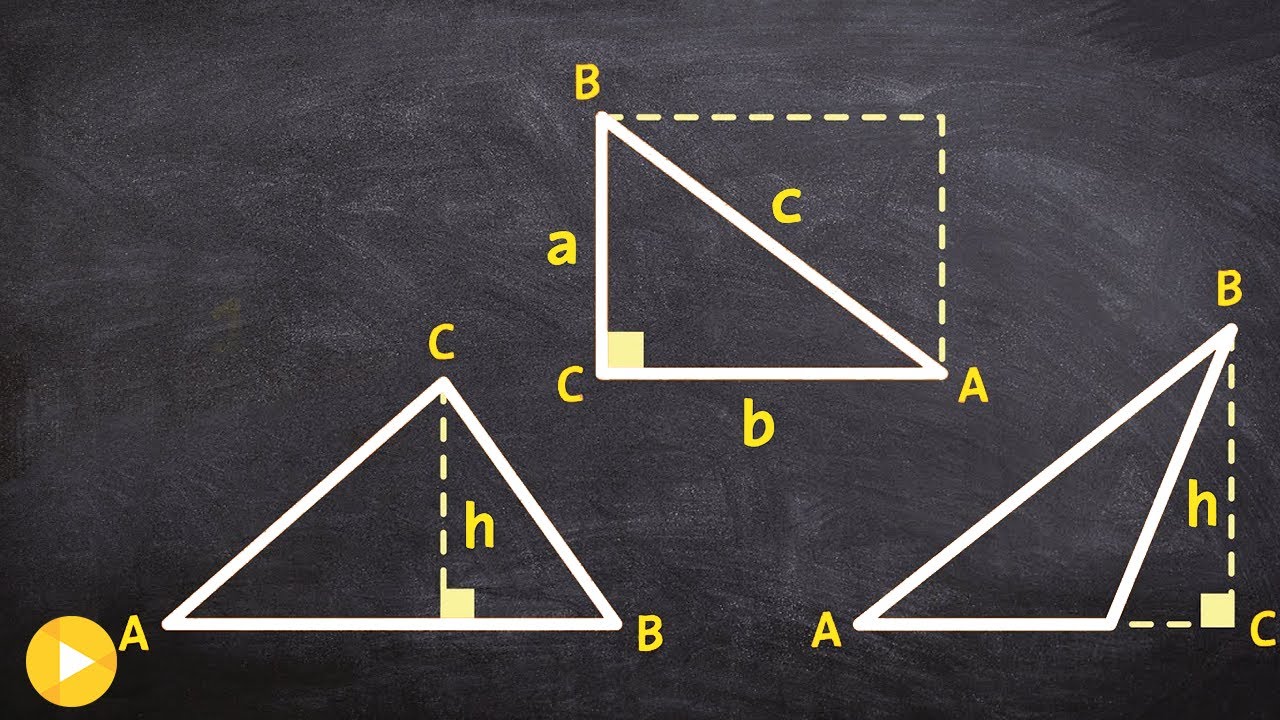
Area Of A Triangle Trigonometry Formula. The area of δabc can be expressed as: In each case, nd h and the area of the triangle.
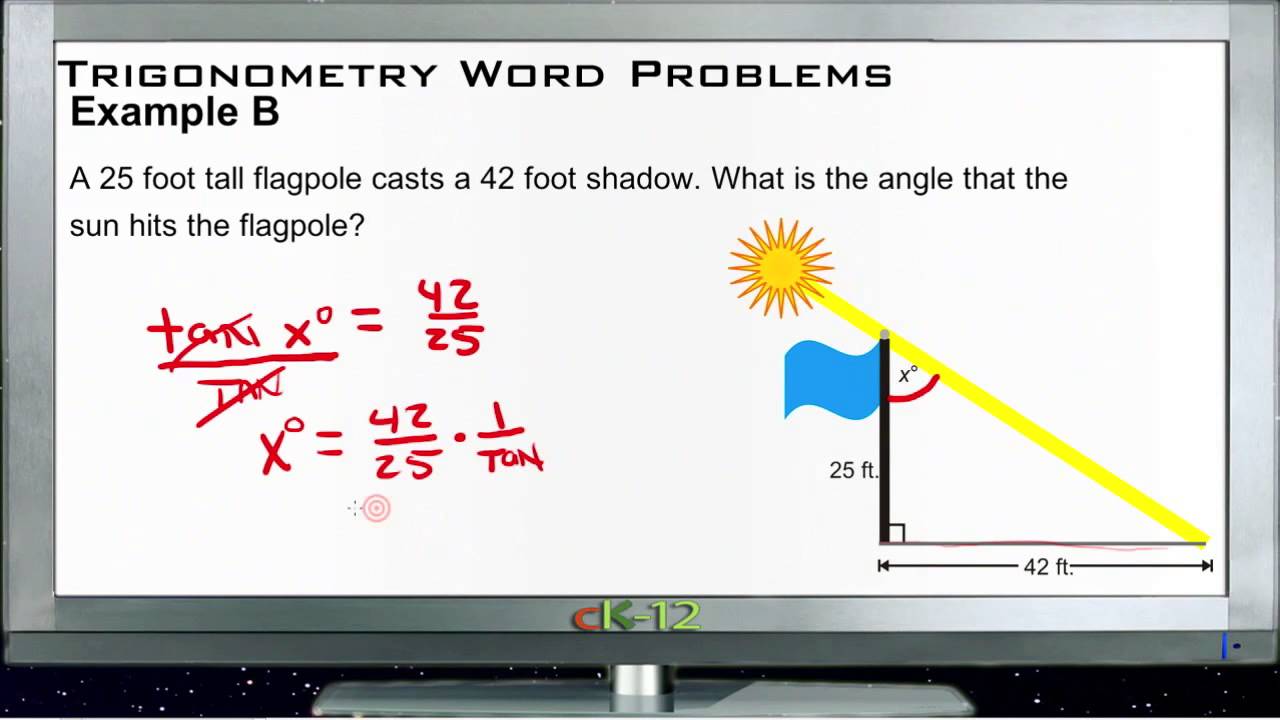
Trigonometry Word Problems Examples (Basic Geometry From youtube.com
Substitute the length of the sides and the angle between them into the formula. The triangle area formula is: Its submitted by organization in the best field.
Trigonometry Word Problems Examples (Basic Geometry
A method for calculating the area of a triangle when you know the lengths of all three sides. The height, h, of the triangle can be expressed as b sin c. Substitute the length of the sides and the angle between them into the formula. We know the base is c, and can work out the height:
Source: youtube.com
Now, if any two sides and the angle between them are given, then the formulas to calculate the area of a triangle is given by: There are several ways to compute the area of a triangle. Its submitted by executive in the best field. Now, using trigonometry, in the right triangle cda, we can state that: We identified it from.
Source: youtube.com
Let a,b,c be the lengths of the sides of a triangle. To find the area of the triangle on the left, substitute the base and the height into the formula for area. Substitute the length of the sides and the angle between them into the formula. Trigonometry, however, provides additional ways to find the area of a triangle using the.
Source: youtube.com
More resources available at www.misterwootube.com The area of δabc can be expressed as: Its submitted by organization in the best field. Let us take a triangle abc, whose vertex angles are ∠a, ∠b, and ∠c, and sides are a,b and c, as shown in the figure below. For instance, there’s the basic formula that the area of a triangle is.
Source: youtube.com
Area = ½ × (c) × (b × sin a) which can be simplified to: While the formula shows the letters b and h , it is actually the pattern of the formula that is important. ½ × a number = 0.5 × a number = a number ÷ 2. Trigonometry, however, provides additional ways to find the area of.
Source: youtube.com
Now, using trigonometry, in the right triangle cda, we can state that: We know the base is c, and can work out the height: X length of base x length of height. You can calculate area of a triangle easily from trigonometry: Area = 0.5 * a * b * sin(γ)
